An angle is the figure formed by two rays, called the sides of the angle, sharing a common endpoint, called the vertex of the angle. Initial side of an angle is the ray where measurement of an angle starts. Terminal side of an angle is the ray where measurement of an angle stops.

Parts of an Angle

The corner point of an angle is called the vertex and the two straight sides are called arms. The angle is the amount of turn between each arm.
Type of Angle Description
Acute Angle an angle that is less than 90°
Right Angle an angle that is 90° exactly
Obtuse Angle an angle that is greater than 90° but less than 180°
Straight Angle an angle that is 180° exactly
Reflex Angle an angle that is greater than 180°
Positive and Negative Angle
The angle is measured by the amount of rotation from the initial side to the terminal side. If measured in a counterclockwise direction the measurement is positive (called positive angle). If measured in a clockwise direction the measurement is negative (called negative angle). (A negative associated with an angle's measure refers to its "direction" of measurement, clockwise.)

Standard Position

An angle is in standard position if its vertex is located at the origin and one ray is on the positive x-axis. The ray on the x-axis is called the initial side and the other ray is called the terminal side. If the terminal side of an angle lies "on" the axes (such as 0º, 90º, 180º, 270º, 360º ), it is called a quadrantal angle. The angle shown at the right is referred to as a Quadrant II angle since its terminal side lies in Quadrant II.
Special angle : 0°, 30°, 45°, 60°, and 90°
DEGREES AND RADIANS
Degrees
We can measure Angles in Degrees.
There are 360 degrees in one Full Rotation (one complete circle around). A Full Circle is 360°.
One complete turn (360°) is equal to 2π radians, so 180° is equal to π radians, or equivalently, the degree is a mathematical constant: 1° = π⁄180.
The Degree Symbol: °
We use a little circle ° following the number to mean degrees. For example 90° means 90 degrees.
Radians
We can measure Angles in Radians.
1 Radian is about 57.2958 degrees. Radian is a pure measure based on the Radius of the circle.
Radians and Degrees
There are π radians in a half circle
And also 180° in a half circle
So π radians = 180°
So, 1 radian = 180°/π = 57.2958° (approximately)
Degrees Radians Radians
(exact) (approx)
0° 0 0
30° π/6 0.524
45° π/4 0.785
60° π/3 1.047
90° π/2 1.571
180° π 3.142
270° 3π/2 4.712
360° 2π 6.283
How to convert
360° = 2π
360° / 2 = 2π / 2
180° / 180° = π / 180°
1° = π / 180°
and
2π radian = 360°
2π radian / 2π = 360° / 2π
1 radians = 180° / π
Example
120° = ... radians
120° = 120° x π/180 radians
= 2/3 π
11/12 π rad = ... °
11/12 π = 180°/π x 11/12 π
= 165°
MULTIPLYING EXPONENTS
There are some rules to solve exponent problems:
Rule 1

If the bases are different but the exponents are the same, then we can combine them.
Example 3^5 x 4^5 = (3 x 4)^5 = 125 = 248832
Rule 2

Dividing different bases can’t be simplified unless the exponents are equal. So, we can divide bases, then count its exponent.
Example 6^3 / 3^3 = (6/3)^3 = 3^3 = 27
Rule 3

We just multiply the exponents.
Example (23)^2 = (2 x 2 x 2)^2 = 8^2 = 64 = 2^6
(23)^2 = (2)^(3x2) = 2^6 = 64
Rule 4

When the bases are the same, then, just adding the exponents.
Example 2^3 x 2^5 = 2^(3+5) = 2^8 = 256
Rule 5

For division with same bases, then subtract exponents.
Example 4^5 / 4^3 = 4^(5-3) = 4^2 = 16
MULTI DIVISION MATH
How to calculate 26 x 31 ?
Standard algorithm for multiplying
26 x 31 = ...
26
31 x
26
78 +
806
We can calculate with other methods:
# Partial Product Method
26 x 31 = ...
26
31 x
6 = 1 x 6
12 = 20 x 1
180 = 30 x 6
600 + = 200 x 3
806
# 26 x 31 = ...
26 = 20 + 5 + 1
then, (20×31) + (5×31) + (1×31) = 620 + 155 + 31 = 806
# Lattice Method
26 x 31 = ...
So, 26 x 31 = 806
Standard algorithm for division
Long Division
133 : 6 = ...
22 Remain 1
6 /133
12 -
13
12 -
1
So, 133 : 6 = 22 1/6
# The other methods
133 : 6 = ...
6 x 10 = 60
6 x 20 = 120
6 x 1 = 6
6 x 21 = 126
6 x 1 = 6
6 x 22 = 132
6×22+1 = 133
So, 133 : 6 = 22 Remain 1
133 : 6 = ...
6 × 10 = 60
6 × 10 = 60
6 × 1 = 6
6 × 1 = 6
(10 + 10 + 1 + 1) = 22
133 = 6 × 22 + 1
So, 133 : 6 = 22 Remain 1
QUADRATICS FORM
Standard form for a quadratic equation:
y = ax2 + bx + c
A quadratic equation is a polynomial function of degree 2, where a, b, and c are all real numbers and a ≠ 0.
Quadratic equations are square. The rate of slope is changing (never same at every point).
Example f(x) = x2 + 4x + 3

Linear equation
y = mx + b
m = slope
b = y-intercept
The gradient is constant and it is in straight line.
Example y = 3x + 1
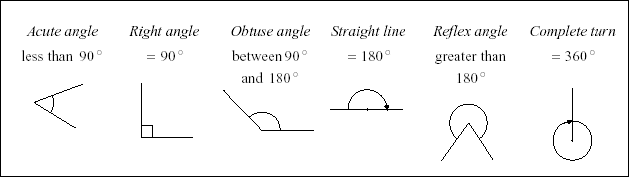


Tidak ada komentar:
Posting Komentar